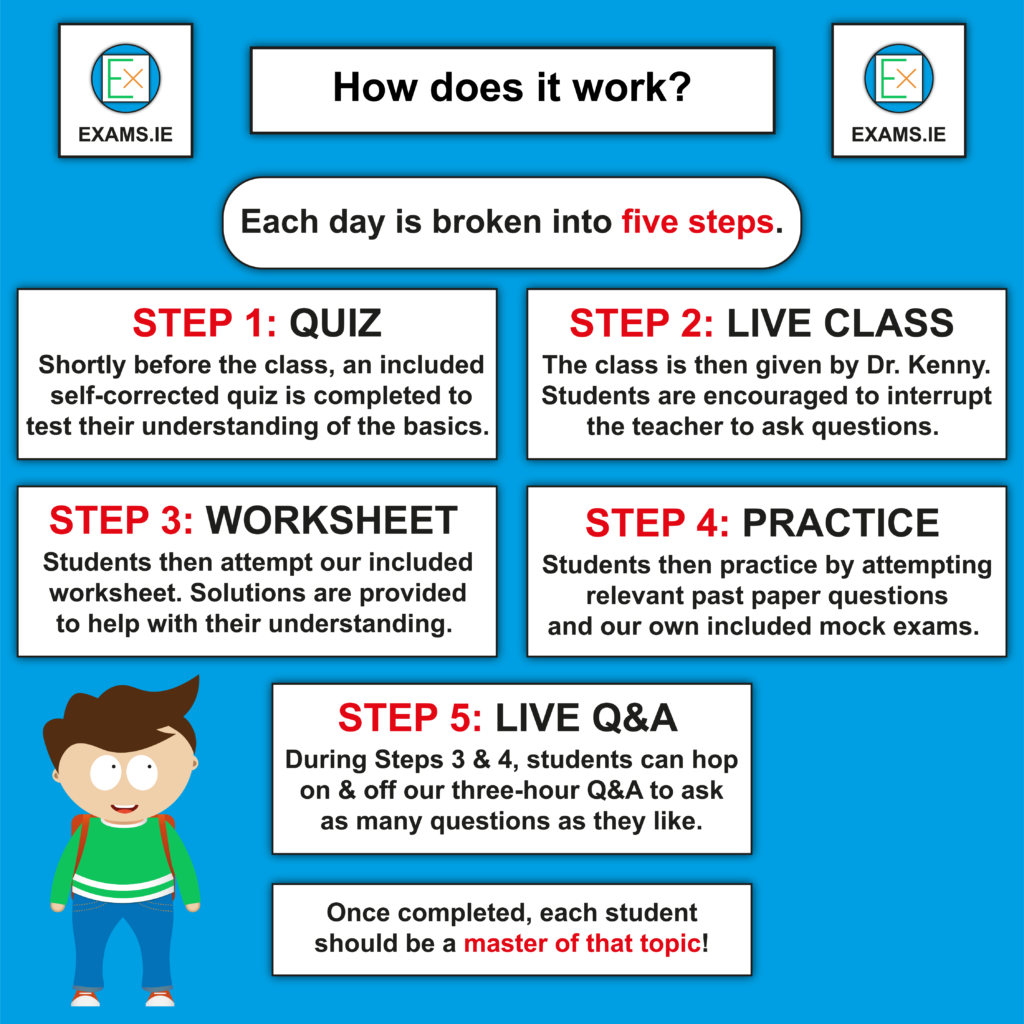
About Lesson
Algebra 1
0/14
Algebra 2
0/16
Trigonometry 1
0/18
Coordinate Geometry of the Line
0/14
Probability 1
0/14
Geometry 1
0/6
Differential Calculus
0/10
Trigonometry 2
0/10
Sequences & Series
0/12
Statistics 1
0/9
Coordinate Geometry of the Circle
0/14
Algebra 3
0/16
Complex Numbers
0/12
Geometry 2
0/4
Integration
0/8
Differential Calculus Applications
0/8
Financial Maths
0/8
Length, Area & Volume
0/6
Probability 2
0/9
Functions
0/15
Statistics 2
0/4
Inferential Statistics
0/6